Centre Of Gravity (O Level)

Centre Of Mass

It is useful in certain calculations to consider an imaginary point in a body of matter where, the total mass or weight of the body seems to concentrate at. The imaginary point is termed as the centre of mass.

Definition: The centre of mass of a body of matter is an imaginary point at which the entire mass of the body seems to act.

Centre Of Gravity

The centre of gravity of a body of matter is an imaginary point at which the entire weight of the body seems to act.

• When a body is in an uniform gravitational field, its centre of gravity is also its centre of mass.
• The location of a body’s centre of gravity may coincide with the geometrical centre of the body, especially in a symmetrically shaped body composed of homogenous material.
• For hollow bodies or irregularly shaped objects, the centre of gravity (or centre of mass) may occur in space at a point external to the physical material (the centre of gravity can lie OUTSIDE the object!) – for example, in the centre of a tennis ball or between the legs of a chair.

Note: Although the term centre of gravity is widely used, the same imaginary point in a body may also be called the centre of mass, since weight and mass are proportional in an uniform gravitational field. Because the centre of mass does not require a gravitational field, many physicists prefer the term centre of mass to centre of gravity.

Example: The centre of mass of an uniform rod is the centre of the rod.

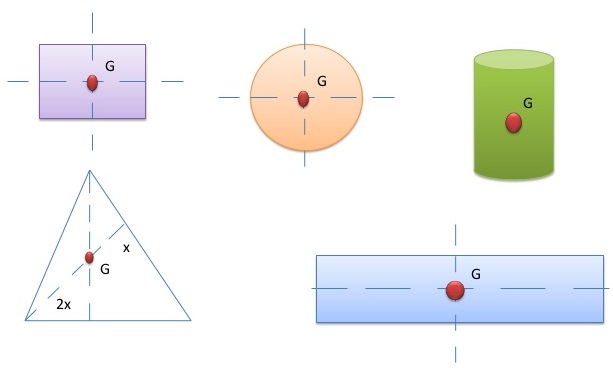
Centre Of Gravity Of Common Shapes:

Determining The Centre Of Gravity Of An Irregular Shaped Lamina

Determining the center of gravity for an irregularly shaped lamina involves a systematic process to locate the point where the entire mass of the object can be considered concentrated. Unlike symmetrical shapes, irregular laminas lack a straightforward geometric center, making the task more intricate. One method to find the center of gravity is through physical experimentation. By suspending the irregular lamina from different points, it is possible to observe the positions at which it comes to rest. The intersection of these suspension points corresponds to the center of gravity, as the lamina remains in equilibrium when suspended from this pivotal location.

Alternatively, mathematical calculations can be employed to find the center of gravity of an irregularly shaped lamina. Breaking down the lamina into simpler geometric shapes and determining the center of gravity for each section allows for a composite calculation. The weighted average of these individual centers of gravity, based on the mass distribution, yields the overall center of gravity for the entire irregular lamina. This method combines mathematical precision with the understanding of how different sections contribute to the overall mass distribution, providing an accurate determination of the center of gravity for irregularly shaped laminas.

Step-By-Step Guide To Determine The Centre Of Gravity Of An Irregular Shaped Lamina

Here’s a step-by-step guide for determining the center of gravity of an irregularly shaped lamina through physical experimentation:

Materials Needed:

1. Irregularly shaped lamina
2. String or thread
3. Support stand
4. Clamp or hook
5. Plumb bob or a small weight
6. Measuring tape or ruler

Procedure:

1. Prepare the Lamina:
   Begin by ensuring the irregularly shaped lamina is clean and free from any obstructions. The accuracy of the experiment relies on the clear observation of the lamina’s behavior when suspended.
2. Set Up the Support Stand:
   Place the support stand on a stable surface. Ensure it has a hook or a clamp that can be adjusted to suspend the lamina.
3. Attach the Lamina:
   Use a string or thread to attach the lamina securely to the hook or clamp on the support stand. Ensure that the lamina is suspended freely and is not in contact with any other surfaces.
4. Observe the Resting Position:
   Allow the lamina to come to rest. Observe the position where it stabilizes. This initial point is a rough estimate of the center of gravity, but further refinement is needed.
5. Adjust the Suspension Point:
   If the lamina is not perfectly balanced, carefully adjust the suspension point on the lamina until it reaches a stable, balanced position. This may require patience and several iterations.
6. Repeat from Different Points:
   Move the suspension point to different locations on the lamina and repeat the process. Take note of each stable position. The center of gravity is likely to be where these points intersect.
7. Calculate the Average:
   If the lamina is irregularly shaped with varying mass distribution, calculate the average position of the stable points. This average position is a more accurate estimate of the center of gravity.
8. Verification:
   To verify the results, repeat the experiment multiple times. Consistency in the observed stable points reinforces the accuracy of the determined center of gravity.

By following these steps, you can conduct a physical experiment to find the center of gravity of an irregularly shaped lamina, providing valuable insights into its mass distribution and equilibrium.

Worked Examples

Example 1

Is the centre of gravity of an object the same whether it is placed near the surface of the Earth or near the surface of the Moon?