Isochoric Process
In an isochoric process, the volume of the system is unchanged (ΔV = 0). Therefore, there is no work done on the system (i.e. W = 0). For such a process, the first law gives:
$$\begin{aligned} \Delta E &= Q \\ &= n C_{v} \Delta T \\ &= \frac{C_{v} (P_{f} – P_{i})V}{R} \end{aligned}$$
This means that the heat input into the system changes its internal energy accordingly.
Isobaric Process
In an isobaric process, the pressure of the system is unchanged. For such a process, the work done by the system takes a simple form:
$$W_{\text{out}} = P(V_{f} – V_{i})$$
Putting the engine cycle together
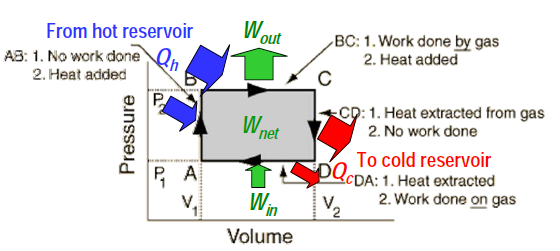Heat engines take in energy in the form of heat, use part of that energy to do useful work, and rejects the remainder of the energy as waste heat. The question is whether there is a minimum amount of waste heat that needs to be rejected, and how much this is?
